Publications 2015-2019

The odd free-surface flows of a colloidal chiral fluid
by V. Soni, S. Magkikiadou, S. Sacanna, D. Bartolo, M. Shelley, and W. Irvine
 in Nature Physics 15, 1188-1194 (2019)

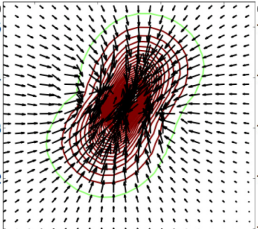
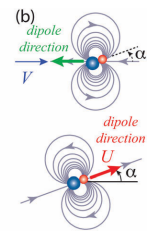
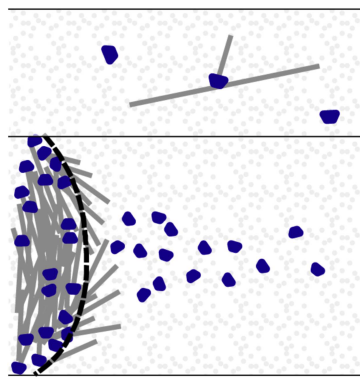
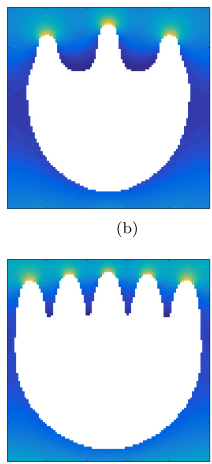
Abstract: In simple fluids, such as water, invariance under parity and time-reversal symmetry imposes that the rotation of constituent ‘atoms’ is determined by the flow and that viscous stresses damp motion. Activation of the rotational degrees of freedom of a fluid by spinning its atomic building blocks breaks these constraints and has thus been the subject of fundamental theoretical interest across classical and quantum fluids. However, the creation of a model liquid that isolates chiral hydrodynamic phenomena has remained experimentally elusive. Here, we report the creation of a cohesive two-dimensional chiral liquid consisting of millions of spinning colloidal magnets and study its flows. We find that dissipative viscous ‘edge-pumping’ is a key and general mechanism of chiral hydrodynamics, driving unidirectional surface waves and instabilities, with no counterpart in conventional fluids. Spectral measurements of the chiral surface dynamics suggest the presence of Hall viscosity, an experimentally elusive property of chiral fluids. Precise measurements and comparison with theory demonstrate excellent agreement with a minimal chiral hydrodynamic model, paving the way for the exploration of chiral hydrodynamics in experiment.

 
The stormy fluid dynamics of the living cell
by D. Needleman and M. Shelley
 in Physics Today 72, 32 (2019)

Abstract: Cell biology has its beginnings in the first observations of cells through primitive microscopes and in the formulation of cell theory, which postulates that cells are the fundamental building blocks of life. Light microscopes showed that the insides of cells contained complex structures, such as nuclei, spindles, and chromosomes. The advent of electron microscopy in the mid 20th century brought the first truly detailed views of cell innards. Images revealed complexity at all observable scales, including cell-spanning networks of polymers, intricate organelles made of membranes, and a variety of micron- to nanometer-sized sacs and granules such as vesicles, lipid droplets, and ribosomes. (For a glossary of cellular components, see the Quick Study by Ned Wingreen, Physics Today, September 2006, page 80.) Those structures are immersed in or part of the aqueous cytoplasm—the cell’s fluidic medium.
Self-straining of actively cross-linked microtubule networks
 by S. Fuerthauer, B. Lemma, P. Foster, S. Ems-McClung, C. Walczak, Z. Dogic, D. Needleman, and M. Shelley
in Nature Physics 15, 1295 (2019)
Abstract: Cytoskeletal networks are foundational examples of active matter and central to self-organized structures in the cell. In vivo, these networks are active and densely crosslinked. Relating their large-scale dynamics to the properties of their constituents remains an unsolved problem. Here, we study an in vitro active gel made from aligned microtubules and XCTK2 kinesin motors. Using photobleaching, we demonstrate that the gel’s aligned microtubules, driven by motors, continually slide past each other at a speed independent of the local microtubule polarity and motor concentration. This phenomenon is also observed, and remains unexplained, in spindles. We derive a general framework for coarse graining microtubule gels crosslinked by molecu-lar motors from microscopic considerations. Using microtubule–microtubule coupling through a force–velocity relationship for kinesin, this theory naturally explains the experimental results: motors generate an active strain rate in regions of changing polarity, which allows microtubules of opposite polarities to slide past each other without stressing the material.
A scalable computational platform for Stokes suspensions
by W. Yan, E. Corona, D. Malhotra, S. Veerapaneni, and M. Shelley
 submitted (2019)
Lattices of hydrodynamically interacting flapping swimmers
by A. Oza, L. Ristroph, and M. Shelley
in Physical Review X 9, 041014 (2019)
Abstract: Fish schools and bird flocks exhibit complex collective dynamics whose self-organization principles are largely unknown. The influence of hydrodynamics on such collectives has been relatively unexplored theoretically, in part due to the difficulty in modeling the temporally long-lived hydrodynamic interactions between many dynamic bodies. We address this through a novel discrete-time dynamical system (iterated map) that describes the hydrodynamic interactions between flapping swimmers arranged in one- and two-dimensional lattice formations. Our 1D results exhibit good agreement with previously published experimental data, in particular predicting the bistability of schooling states and new instabilities that can be probed in experimental settings. For 2D lattices, we determine the formations for which swimmers optimally benefit from hydrodynamic interactions. We thus obtain the following hierarchy: while a side-by-side single-row “phalanx” formation offers a small improvement over a solitary swimmer, 1D in-line and 2D rectangular lattice formations exhibit substantial improvements, with the 2D diamond lattice offering the largest hydrodynamic benefit. Generally, our self-consistent modeling framework may be broadly applicable to active systems in which the collective dynamics is primarily driven by a fluid-mediated memory.
Rotating membrane inclusions crystallize through hydrodynamics and steric interactions
by N. Oppenheimer, D. Stein, and M. Shelley
 in Physical Review Letters 123, 148101 (2019)
Abstract: We show that rotating membrane inclusions can crystallize due to combined hydrodynamic and steric interactions. Alone, steric repulsion of unconfined particles, even with thermal fluctuations, does not lead to crystallization, nor do rotational hydrodynamic interactions which allow only a marginally stable lattice.Hydrodynamic interactions enable particles to explore states inaccessible to a nonrotational system, yet, unlike Brownian motion, Hamiltonian conservation confines the ensemble which, when combined with steric interactions, anneals into a stable crystal state.
Relating rheotaxis and hydrodynamic actuation using asymmetric gold-platinum phoretic rods
by Q. Brosseau, F. Balboa Usabiaga, E. Lushi, Y. Wu, L. Ristroph, J. Zhang, M. Ward, and M. Shelley
in Physical Review Letters 123, 178004 (2019)
Abstract: We explore the behavior of micron-scale autophoretic Janus (Au=Pt) rods, having various Au=Pt length ratios, swimming near a wall in an imposed background flow. We find that their ability to robustly orient and move upstream, i.e., to rheotax, depends strongly on the Au=Pt ratio, which is easily tunable insynthesis. Numerical simulations of swimming rods actuated by a surface slip show a similar rheotactic tunability when varying the location of the surface slip versus surface drag. The slip location determines whether swimmers are pushers (rear actuated), pullers (front actuated), or in between. Our simulations and modeling show that pullers rheotax most robustly due to their larger tilt angle to the wall, which makes them responsive to flow gradients. Thus, rheotactic response infers the nature of difficult to measure flow fields of an active particle, establishes its dependence on swimmer type, and shows how Janus rods can be tuned for flow responsiveness.
Coarse-graining the dynamics of immersed and driven fiber assemblies
by D. Stein and M. Shelley
 in Physical Review Fluids 4, 073302 (2019)
Abstract: An important class of fluid-structure problems involve the dynamics of ordered arrays of immersed,flexible fibers. While specialized numerical methods have been developed to study fluid-fiber systems, they become infeasible when there are many, rather than a few, fibers present, nor do these methods lend themselves to analytical calculation. Here, we introduce a coarse-grained continuum model, based on local-slender body theory, for elastic fibers immersed in a viscous Newtonian fluid.It takes the form of an anisotropic Brinkman equation whose skeletal drag is coupled to elastic forces...
Dynamics of flexible fibers in viscous flows and fluids
by O. du Roure, A. Lindner, E. Nazockdast, and M. Shelley
in Annual Reviews of Fluid Mechanics 51, 539-572 (2019)
Abstract: The dynamics and deformations of immersed flexible fibers are at the heart of important industrial and biological processes, induce peculiar mechanical and transport properties in the fluids that contain them, and are the basis for novel methods of flow control. Here we focus on the low–Reynolds number regime where advances in studying these fiber–fluid systems have been especially rapid. On the experimental side, this is due to new methods of fiber synthesis, microfluidic flow control, and microscope-based tracking measurement techniques. Likewise, there have been continuous improvements in the specialized mathematical modeling and numerical methods needed to capture the interactions of slender flexible fibers with flows, boundaries, and each other.
Calculating the collision stress in assemblies of active spherocylinders: applications of a fast and generic geometric method
by W. Yan, H. Zhang, M. Shelley
in Journal of Chemical Physics 150, 064109 (2019)

Abstract: In this work we provide a solution to the problem of computing collision stress in particle-tracking simulations. First, a formulation for the collision stress between particles is derived as an extension of the virial stress formula to general-shaped particles with uniform or non-uniform density. Second, we describe a collision-resolution algorithm based on geometric constraint minimization which eliminates the stiff pairwise potentials in traditional methods. The method is validated with a comparison to the equation of state of Brownian spherocylinders. We demonstrate the application of this method in several emerging problems of soft active matter.
 
 Current approaches for the analysis of spindle organization
by S. Redemann, S. Fürthauer, M. Shelley, and Th. Müller-Reichert
in Current Opinion in Structural Biology, 58, 269 (2019).

Abstract: The organization of microtubules in spindles is complex and not fully understood. Here we report on current advances in generating 3D reconstructions of staged spindles by serial-section electron tomography, exemplified by the first mitotic spindle in early Caenorhabditis elegans embryo. We then review how advances in correlative light microscopy and quantitative electron tomography enable the development of theory and stochastic simulations, which describe how the microtubule organization in spindles emerges from their dynamics. We show how theory and simulations can be used to address long-standing questions in cell division research, advancing the field beyond a pure structural description of microtubules in spindles.
Active matter invasion of a viscous fluid and a no-flow theorem
by C. Miles, A. Evans, M. Shelley, and S. Spagnolie
in Physical Review Letters 122, 098002 (2019)

Abstract: We investigate the dynamics of a dilute suspension of hydrodynamically interacting motile or immotile stress-generating swimmers or particles as they invade a surrounding viscous fluid. Colonies of aligned pusher particles are shown to elongate in the direction of particle orientation and undergo a cascade of transverse concentration instabilities, governed at small times by an equation which also describes the Saffman-Taylor instability in a Hele-Shaw cell, or Rayleigh-Taylor instability in two-dimensional flow through a porous medium. Thin sheets of aligned pusher particles are always unstable, while sheets of aligned puller particles can either be stable (immotile particles), or unstable (motile particles) with a growth rate which is non-monotonic in the force dipole strength. We also prove a surprising “no-flow theorem”: a distribution initially isotropic in orientation loses isotropy immediately but in such a way that results in no fluid flow everywhere and for all time.
A compact Eulerian representation of axisymmetric inviscid vortex sheet dynamics
 by A. Pesci, R. Goldstein and M. Shelley
to appear in Communications in Pure and Applied Mathematics (2019)
The evolution of large-scale modeling of Monkey primary visual cortex, V1: steps towards understanding cortical functioning
 by L.-S. Young, L. Tao, M. Shelley, R. Shapley, A. Rangan, and D. McLaughlin
in Communications in Mathematical Sciences 5, 1387 (2019)
 
 From cytoskeletal assemblies to living materials
 by P. Foster, S. Fürthauer, M. Shelley, D. Needleman
in Current Opinion in Cell Biology 56, 109 (2019)
Abstract: Many subcellular structures contain large numbers of cytoskeletal filaments. Such assemblies underlie much of cell division, motility, signaling, metabolism, and growth. Thus, understanding cell biology requires understanding the properties of networks of cytoskeletal filaments. While there are well established disciplines in biology dedicated to studying isolated proteins — their structure (Structural Biology) and behaviors (Biochemistry) — it is much less clear how to investigate, or even just describe, the structure and behaviors of collections of cytoskeletal filaments. One approach is to use methodologies from Mechanics and Soft Condensed Matter Physics, which have been phenomenally successful in the domains where they have been traditionally applied. From this perspective, collections of cytoskeletal filaments are viewed as materials, albeit very complex, ‘active’ materials, composed of molecules which use chemical energy to perform mechanical work.
 Extensile motor activity drives coherent motions in a model of interphase chromatin
by S. Saintillan, M. Shelley, and A. Zidovska
in Proceedings of the National Academy of Science 115, 11442 (2018)
Abstract: The 3D spatiotemporal organization of the human genome inside the cell nucleus remains a major open question in cellular biology. In the time between two cell divisions, chromatin—the functional form of DNA in cells—fills the nucleus in its uncondensed polymeric form. Recent in vivo imaging experiments reveal that the chromatin moves coherently, having displacements with long-ranged correlations on the scale of micrometers and lasting for seconds. To elucidate the mechanism(s) behind these motions, we develop a coarse-grained active polymer model where chromatin is represented as a confined flexible chain acted upon by molecular motors that drive fluid flows by exerting dipolar forces on the system. Numerical simulations of this model account for steric and hydrodynamic interactions as well as internal chain mechanics. These demonstrate that coherent motions emerge in systems involving extensile dipoles and are accompanied by large-scale chain reconfigurations and nematic ordering. Comparisons with experiments show good qualitative agreement and support the hypothesis that self-organizing long-ranged hydrodynamic couplings between chromatin-associated active motor proteins are responsible for the observed coherent dynamics.  
 
Universal image systems for non-periodic and periodic Stokes flows above a wall
by W. Yan and M. Shelley
in Journal of Computational Physics 375, 263–270 (2018)

 Abstract: It is well-known that by placing judiciously chosen image point forces and doublets to the Stokeslet above a flat wall, the no-slip boundary condition can be conveniently imposed on the wall Blake (1971) [8]. However, to further impose periodic boundary conditions on directions parallel to the wall usually involves tedious derivations because single or double periodicity in Stokes flow may require the periodic unit to have no net force, which is not satisfied by the well-known image system. In this work we present a force-neutral image system. This neutrality allows us to represent the Stokes image system in a universal formulation for non-periodic, singly periodic and doubly periodic geometries. This formulation enables the black-box style usage of fast kernel summation methods. We demonstrate the efficiency and accuracy of this new image method with the periodic kernel independent fast multipole method in both non-periodic and periodic geometries. We then extend this new image system to other widely used Stokes fundamental solutions, including the Laplacian of the Stokeslet and the Rotne–Prager–Yamakawa tensor. 
 
Nonlinear concentration patterns and bands in autochemotactic suspensions
by E. Lushi, R. Goldstein, and M. Shelley 
in Physical Review E 98, 052411 (2018)
Abstract: In suspensions of microorganisms, pattern formation can arise from the interplay of chemotaxis and the fluid flows collectively generated by the organisms themselves. Here we investigate the resulting pattern formation in square and elongated domains in the context of two distinct models of locomotion in which the chemoattractant dynamics is fully coupled to the fluid flows and swimmer motion. Analyses for both models reveal an aggregative instability due to chemotaxis, independent of swimmer shape and type, and a hydrodynamic instability for “pusher” swimmers. We discuss the similarities and differences between the models. Simulations reveal a critical length scale of the swimmer aggregates and this feature can be utilized to stabilize swimmer concentration patterns into quasi-one-dimensional bands by varying the domain size. These concentration bands transition to traveling pulses under an external chemoattractant gradient, as observed in experiments with chemotactic bacteria. 
 
Equilibrium shapes and their stability for liquid films in fast flows
by L. Ganedi, A. Oza, M. Shelley, and L. Ristroph
in Physical Review Letters 121, 094501 (2018)

Abstract:We study how a suspended liquid film is deformed by an external flow en route to forming a bubble through experiments and a model. We identify a family of nonminimal but stable equilibrium shapes for flow speeds up to a critical value beyond which the film inflates unstably, and the model accounts for the observed nonlinear deformations and forces. A saddle-node or fold bifurcation in the solution diagram suggests that bubble formation at high speeds results from the loss of equilibrium and at low speeds from the loss of stability for overly inflated shapes. 
 
Flexibly imposing periodicity in kernel independent FMM: A Multipole-To-Local operator approach
by W. Yan and M. Shelley
in Journal of Computational Physics 355, 214-232 (2018)

 Abstract: An important but missing component in the application of the kernel independent fast multipole method (KIFMM) is the capability for flexibly and efficiently imposing singly, doubly, and triply periodic boundary conditions. In most popular packages such periodicities are imposed with the hierarchical repetition of periodic boxes, which may give an incorrect answer due to the conditional convergence of some kernel sums. Here we present an efficient method to properly impose periodic boundary conditions using a near-far splitting scheme. The near-field contribution is directly calculated with the KIFMM method, while the far-field contribution is calculated with a multipole-to-local (M2L) operator which is independent of the source and target point distribution. The M2L operator is constructed with the far-field portion of the kernel function to generate the far-field contribution with the downward equivalent source points in KIFMM. This method guarantees the sum of the near-field & far-field converge pointwise to results satisfying periodicity and compatibility conditions. The computational cost of the far-field calculation observes the same O ( N ) complexity as FMM and is designed to be small by reusing the data computed by KIFMM for the near-field. The far-field calculations require no additional control parameters, and observes the same theoretical error bound as KIFMM. We present accuracy and timing test results for the Laplace kernel in singly periodic domains and the Stokes velocity kernel in doubly and triply periodic domains. 
Bistability in the synchronization of actuated microfilaments
by H. Guo, L. Fauci, M. Shelley, and E. Kanso
in Journal of Fluid Mechanics 836, 304-323 (2018)
Abstract:The cellular cytoskeleton is an active material, driven out of equilibrium by molecular motor proteins. It is not understood how the collective behaviors of cytoskeletal networks emerge from the properties of the network’s constituent motor proteins and filaments. Here we present experimental results on networks of stabilized microtubules in Xenopus oocyte extracts, which undergo spontaneous bulk contraction driven by the motor protein dynein, and investigate the effects of varying the initial microtubule density and length distribution. We find that networks contract to a similar final density, irrespective of the length of microtubules or their initial density, but that the contraction timescale varies with the average microtubule length. To gain insight into why this microscopic property influences the macroscopic network contraction time, we developed simulations where microtubules and motors are explicitly represented. The simulations qualitatively recapitulate the variation of contraction timescale with microtubule length, and allowed stress contributions from different sources to be estimated and decoupled.
 
Activity-induced instability in 1D microfluidic crystals
by A.C. Hou Tsang, M. Shelley, and E. Kanso
in Soft Matter 14, 945 (2018)
Abstract: One-dimensional crystals of passively-driven particles in microfluidic channels exhibit collective vibrational modes reminiscent of acoustic ‘phonons’. These phonons are induced by the long-range hydrodynamic interactions among the particles and are neutrally stable at the linear level. Here, we analyze the effect of particle activity – self-propulsion – on the emergence and stability of these phonons. We show that the direction of wave propagation in active crystals is sensitive to the intensity of the background flow. We also show that activity couples, at the linear level, transverse waves to the particles’ rotational motion, inducing a new mode of instability that persists in the limit of large background flow, or, equivalently, vanishingly 

Measuring and modeling polymer gradients argues that spindle microtubules regulate their own nucleation
by B. Kaye, O. Stiehl, P. Foster, M. Shelley, D. Needleman, and S. Fuerthauer
in New Journal of Physics 20, 055012 (2018)

Abstract:Spindles are self-organized microtubule-based structures that segregate chromosomes during cell division. The mass of the spindle is controlled by the balance between microtubule turnover and nucleation. The mechanisms that control the spatial regulation of microtubule nucleation remain poorly understood. While previous work found that microtubule nucleators bind to pre-existing microtubules in the spindle, it is still unclear whether this binding regulates the activity of those nucleators. Here we use a combination of experiments and mathematical modeling to investigate this issue. We measured the concentration of microtubules and soluble tubulin in and around the spindle. We found a very sharp decay in the concentration of microtubules at the spindle interface. This is inconsistent with a model in which the activity of nucleators is independent of their association with microtubules but consistent with a model in which microtubule nucleators are only active when bound to pre-existing microtubules. This argues that the activity of microtubule nucleators is greatly enhanced when bound to pre-existing microtubules. Thus, microtubule nucleators are both localized and activated by the microtubules they generate.  

 
Directed migration of microscale swimmers by an array of shaped obstacles: modeling and shape optimization
by J. Tong and M. Shelley
in SIAM Journal of Applied Mathematics 78, 2370-2392 (2018)
Abstract: Achieving macroscopic directed migration of microscale swimmers in a fluid is an important step towards utilizing their autonomous motion. It has been experimentally shown that directed motion can be induced, without any external fields, by certain geometrically asymmetric obstacles due to interaction between their boundaries and the swimmers. In this paper, we propose a kinetic-type model to study swimming and directional migration of microscale bimetallic rods in a periodic array of posts with noncircular cross-sections. Both rod position and orientation are taken into account; rod trapping and release on the post boundaries are modeled by empirically characterizing curvature and orientational dependence of the boundary absorption and desorption. Intensity of the directed rod migration, which we call the normalized net flux, is then defined and computed given the geometry of the post array. We numerically study the effect of post spacings on the flux; we also apply shape optimization to find better post shapes that can induce stronger flux. Inspired by preliminary numerical results on two candidate posts, we perform an approximate analysis on a simplified model to show the key geometric features that a good post should have. Based on this, three new candidate shapes are proposed which give rise to large fluxes. This approach provides an effective tool and guidance for experimentally designing new devices that induce strong directed migration of microscale swimmers. 
 
Connecting macroscopic dynamics with microscopic properties in active microtubule network contraction
by P. Foster, W. Yan, S. Fuerthauer, M. Shelley, and D. Needleman
in New Journal of Physics 19, 125011 (2017)
Abstract:The cellular cytoskeleton is an active material, driven out of equilibrium by molecular motor proteins. It is not understood how the collective behaviors of cytoskeletal networks emerge from the properties of the network’s constituent motor proteins and filaments. Here we present experimental results on networks of stabilized microtubules in Xenopus oocyte extracts, which undergo spontaneous bulk contraction driven by the motor protein dynein, and investigate the effects of varying the initial microtubule density and length distribution. We find that networks contract to a similar final density, irrespective of the length of microtubules or their initial density, but that the contraction timescale varies with the average microtubule length. To gain insight into why this microscopic property influences the macroscopic network contraction time, we developed simulations where microtubules and motors are explicitly represented. The simulations qualitatively recapitulate the variation of contraction timescale with microtubule length, and allowed stress contributions from different sources to be estimated and decoupled.
 
Guiding microscale swimmers using teardrop-shaped posts
 by M. Davies Wykes, X. Zhong, J. Tong, T. Adachi, Y. Liu, L. Ristroph, M. Ward, M. Shelley, and J. Zhang, in Soft Matter 13, 4681-4688 (2017).
Abstract: The swimming direction of biological or artificial microscale swimmers tends to be randomised over long time-scales by thermal fluctuations. Bacteria use various strategies to bias swimming behaviour and achieve directed motion against a flow, maintain alignment with gravity or travel up a chemical gradient. Herein, we explore a purely geometric means of biasing the motion of artificial nanorod swimmers. These artificial swimmers are bimetallic rods, powered by a chemical fuel, which swim on a substrate printed with teardrop-shaped posts. The artificial swimmers are hydrodynamically attracted to the posts, swimming alongside the post perimeter for long times before leaving. The rods experience a higher rate of departure from the higher curvature end of the teardrop shape, thereby introducing a bias into their motion. This bias increases with swimming speed and can be translated into a macroscopic directional motion over long times by using arrays of teardrop-shaped posts aligned along a single direction. This method provides a protocol for concentrating swimmers, sorting swimmers according to different speeds, and could enable artificial swimmers to transport cargo to desired locations.
 
Analytical structure, dynamics, and reduction of a kinetic model of an active fluid
 by T. Gao, M. Betterton, A. Huang, and M. Shelley
in Physical Review Fluids 2, 093302 (2017).
Abstract:We analyze one of the simplest active suspensions with complex dynamics: a suspension of immotile “extensor” particles that exert active extensile dipolar stresses on the fluid in which they are immersed. This is relevant to several experimental systems, such as recently studied tripartite rods that create extensile flows by consuming a chemical fuel. We first describe the system through a Doi-Onsager kinetic theory based on microscopic modeling. This theory captures the active stresses produced by the particles that can drive hydrodynamic instabilities, as well as the steric interactions of rodlike particles that lead to nematic alignment. This active nematic system yields complex flows and disclination defect dynamics very similar to phenomenological Landau–deGennes Q-tensor theories for active nematic fluids, as well as by more complex Doi-Onsager theories for polar microtubule–motor-protein systems. We apply the quasiequilibrium Bingham closure, used to study suspensions of passive microscopic rods, to develop a nonstandard Q-tensor theory. We demonstrate through simulation that this BQ-tensor theory gives an excellent analytical and statistical accounting of the suspension's complex dynamics, at a far reduced computational cost. Finally, we apply the BQ-tensor model to study the dynamics of extensor suspensions in circular and biconcave domains. In circular domains, we reproduce previous results for systems with weak nematic alignment, but for strong alignment we find unusual dynamics with activity-controlled defect production and absorption at the boundaries of the domain. In biconcave domains, a Fredericks-like transition occurs as the width of the neck connecting the two disks is varied.
 
A computational model of the flight dynamics and aerodynamics of a jellyfish-like flying machine
by F. Fang, K. Ho, L. Ristroph, and M. Shelley
in Journal of Fluid Mechanics 819, 621-655 (2017).
Abstract: We explore theoretically the aerodynamics of a recently fabricated jellyfish-like flying machine (Ristroph & Childress, J. R. Soc. Interface , vol. 11 (92), 2014, 20130992). This experimental device achieves flight and hovering by opening and closing opposing sets of wings. It displays orientational or postural flight stability without additional control surfaces or feedback control. Our model ‘machine’ consists of two mirror-symmetric massless flapping wings connected to a volumeless body with mass and moment of inertia. A vortex sheet shedding and wake model is used for the flow simulation. Use of the fast multipole method allows us to simulate for long times and resolve complex wakes. We use our model to explore the design parameters that maintain body hovering and ascent, and investigate the performance of steady ascent states. We find that ascent speed and efficiency increase as the wings are brought closer, due to a mirror-image ‘ground-effect’ between the wings. Steady ascent is approached exponentially in time, which suggests a linear relationship between the aerodynamic force and ascent speed. We investigate the orientational stability of hovering and ascent states by examining the flyer’s free response to perturbation from a transitory external torque. Our results show that bottom-heavy flyers (centre of mass below the geometric centre) are capable of recovering from large tilts, whereas the orientation of the top-heavy flyers diverges. These results are consistent with the experimental observations in Ristroph & Childress ( J. R. Soc. Interface , vol. 11 (92), 2014, 20130992), and shed light upon future designs of flapping-wing micro aerial vehicles that use jet-based mechanisms.
 
Fast accurate methods for simulating fiber suspensions applied to cellular mechanics
by E. Nazockdast, A. Rahimian, D. Zorin, and M. Shelley
in Journal of Computational Physics 329, 173-209 (2017).
Abstract: We present a novel platform for the large-scale simulation of three-dimensional fibrous structures immersed in a Stokesian fluid and evolving under confinement or in free-space in three dimensions. One of the main motivations for this work is to study the dynamics of fiber assemblies within biological cells. For this, we also incorporate the key biophysical elements that determine the dynamics of these assemblies, which include the polymerization and depolymerization kinetics of fibers, their interactions with molecular motors and other objects, their flexibility, and hydrodynamic coupling. This work, to our knowledge, is the first technique to include many-body hydrodynamic interactions (HIs), and the resulting fluid flows, in cellular assemblies of flexible fibers....
 
 Cytoplasmic flows as signatures for the mechanics of mitotic positioning
by E. Nazockdast, A. Rahimian, D. Needleman, and M. Shelley
in Molecular Biology of the Cell 28, 3261-3270 (2017).
Abstract: The proper positioning of the mitotic spindle is crucial for asymmetric cell division and generating cell diversity during development. Proper position in the single-cell embryo of Caenorhabditis elegans is achieved initially by the migration and rotation of the pronuclear complex (PNC) and its two associated centrosomal arrays of microtubules (MTs). We present here the first systematic theoretical study of how these O(1000) centrosomal microtubules (MTs) interact through the immersing cytoplasm, the cell periphery and PNC, and with each other, to achieve proper position. This study is made possible through our development of a highly efficient and parallelized computational framework that accounts explicitly for long-ranged hydrodynamic interactions (HIs) between the MTs, while also capturing their flexibility, dynamic instability, and interactions with molecular motors and boundaries. First, we show through direct simulation that previous estimates of the PNC drag coefficient, based on either ignoring or partially including HIs, lead to misprediction of the active forces and time-scales of migration. We then directly study the dynamics of PNC migration under various force-transduction models, including the pushing or pulling of MTs at the cortex, and the pulling of MTs by cytoplasmically-bound force generators. While achieving proper position and orientation on physiologically reasonable time-scales does not uniquely choose a model, we find that each model produces a different signature in its induced cytoplasmic flow and MT conformations. We suggest then that cytoplasmic flows and MT conformations can be used to differentiate between mechanisms and to determine their contribution to the migration process.  
 
C. elegans chromosomes connect to centrosomes by anchoring into the spindle network
by S. Redemann et al
in Nature Communications 8, 15288 (2017).
Abstract: The mitotic spindle ensures the faithful segregation of chromosomes. To discover the nature of the crucial centrosome-to-chromosome connection during mitosis, we combined the first large-scale serial electron tomography of whole mitotic spindles in early C. elegans embryos with live-cell imaging. Using tomography, we reconstructed the positions of all microtubules in 3D, and identified their plus- and minus-ends. We classified them as kinetochore (KMTs), spindle (SMTs), or astral microtubules (AMTs) according to their positions, and quantified distinct properties of each class. While our light microscopy and mutant studies show that microtubules are nucleated from the centrosomes, we find only a few KMTs are directly connected to the centrosomes. Indeed, by quantitatively analysing several models of microtubule growth, we conclude that minus-ends of KMTs have selectively detached and depolymerized from the centrosome. In toto, our results show that the connection between centrosomes and chromosomes is mediated by an anchoring into the entire spindle network and that any direct connections through KMTs are few and likely very transient.
 
Forces positioning the mitotic spindle in the cell; Theories, and now experiments
by H. Wu, E. Nazockdast, M. Shelley, and D. Needleman
in BioEssays 39, 1600212 (2017).
Abstract: The position of the spindle determines the position of the cleavage plane, and is thus crucial for cell division. Although spindle positioning has been extensively studied, the underlying forces ultimately responsible for moving the spindle remain poorly understood. A recent pioneering study by Garzon-Coral et al. uses magnetic tweezers to perform the first direct measurements of the forces involved in positioning the mitotic spindle. Combining this with molecular perturbations and geometrical effects, they use their data to argue that the forces that keep the spindle in its proper position for cell division arise from astral microtubules growing and pushing against the cell's cortex. Here, we review these ground-breaking experiments, the various biomechanical models for spindle positioning that they seek to differentiate, and discuss new questions raised by these measurements.

Dynamic self-assembly of microscale rotors and swimmers
by M. Davies Wykes, J. Palacci, T. Adachi, L. Ristroph, X. Zhong, M. Ward, J. Zhang, and M. Shelley
in Soft Matter 12, 4584-4589 (2016).

Abstract: Biological systems often involve the self-assembly of basic components into complex and functioning structures. Artificial systems that mimic such processes can provide a well-controlled setting to explore the principles involved and also synthesize useful micromachines. Our experiments show that immotile, but active, components self-assemble into two types of structure that exhibit the fundamental forms of motility: translation and rotation. Specifically, micron-scale metallic rods are designed to induce extensile surface flows in the presence of a chemical fuel; these rods interact with each other and pair up to form either a swimmer or a rotor. Such pairs can transition reversibly between these two configurations, leading to kinetics reminiscent of bacterial run-and-tumble motion.

Elastic fibers in flows
by A. Lindner and M. Shelley
in Fluid-structure interactions at low Reynolds numbers, eds. C. Duprat and H. A. Stone, Royal Society of Chemistry (2016)

Introduction:  A very common class of fluid-structure interaction problems involves the dynamics of flexible fibers immersed in a Stokesian fluid. In biology this arises in modeling the flagellae or cilia involved in micro-organismal locomotion and mucal transport, in determining the shape of biofilm streamers, and in understanding how biopolymers such as microtubules respond to the active coupling afforded by motor proteins. In engineering it arises in the paper processing industry, where wood pulp suspensions can show the abrupt appearance of normal stress differences, and in micro-fluidic engineering where flow control using flexible particles has lately been explored. Flow induced buckling of fibers is an important determinant on fiber transport in those flows, as well as for the fluid mechanical stresses that develop...

The dynamics of microtubule/motor-protein assemblies in biology and physics
by M. Shelley
In Annual Reviews of Fluid Mechanics 48, 487-506 (2016)

Abstract: Many important processes in the cell are mediated by stiff microtubule polymers and the active motor proteins moving upon them. This includes the transport of subcellular structures (nuclei, chromosomes, organelles),  and the self-assembly and positioning of the mitotic spindle. Very little is yet understood of these processes but they all present fascinating problems in fluid/structure interactions. Microtubules and motor proteins are also the building blocks of new "bio-synthetic" active suspensions driven by motor-protein activity. These reduced systems can probed, and modeled, more easily than the fully biological ones and show their own aspects of self-assembly and complex dynamics. I will review recent work modeling such systems as  uid/structure interaction problems, and as multiscale complex  fluids.

Active contraction of microtubule networks
by P. Foster, S. Furthauer, M. Shelley, and D. Needleman
in eLife, 10837 (2015)

Abstract: Many cellular processes are driven by cytoskeletal assemblies. It remains unclear how cytoskeletal filaments and motor proteins organize into cellular scale structures and how molecular properties of cytoskeletal components affect the large scale behaviors of these systems. Here we investigate the self-organization of stabilized microtubules in Xenopus oocyte extracts and find that they can form macroscopic networks that spontaneously contract. We propose that these contractions are driven by the clustering of microtubule minus ends by dynein. Based on this idea, we construct an active fluid theory of network contractions which predicts a dependence of the timescale of contraction on initial network geometry, a development of density inhomogeneities during contraction, a constant final network density, and a strong influence of dynein inhibition on the rate of contraction, all in quantitative agreement with experiments. These results demonstrate that the motor-driven clustering of filament ends is a generic mechanism leading to contraction

Multiscale modeling and simulation of microtubule�motor-protein assemblies
by T. Gao, R. Blackwell, M. Glaser, D. Betterton, and M. Shelley
In Physical Review E 92, 062709 (2015)

Abstract: Microtubules and motor proteins self-organize into biologically important assemblies including the mitotic spindle and the centrosomal microtubule array. Outside of cells, microtubule-motor mixtures can form novel active liquid-crystalline materials driven out of equilibrium by adenosine triphosphate�consuming motor proteins. Microscopic motor activity causes polarity-dependent interactions between motor proteins and microtubules, but how these interactions yield such larger-scale dynamical behavior such as complex flows and defect dynamics is not well understood. We develop a multiscale theory for microtubule-motor systems in which Brownian dynamics simulations of polar microtubules driven by motors are used to study microscopic organization and stresses created by motor-mediated microtubule interactions. We identify polarity-sorting and crosslink tether relaxation as two polar-specific sources of active destabilizing stress. We then develop a continuum Doi-Onsager model that captures polarity sorting and the hydrodynamic flows generated by these polar-specific active stresses. In simulations of active nematic flows on immersed surfaces, the active stresses drive turbulent flow dynamics and continuous generation and annihilation of disclination defects. The dynamics follow from two instabilities, and accounting for the immersed nature of the experiment yields unambiguous characteristic length and time scales. When turning off the hydrodynamics in the Doi-Onsager model, we capture formation of polar lanes as observed in the Brownian dynamics simulation.


Hydrodynamic schooling of flapping swimmers
by A. Becker, H. Masoud, J. Newbolt, M. Shelley, and L. Ristroph
in Nature Communications 6, 8514 (2015)

Abstract: Fish schools and bird flocks are fascinating examples of collective behaviours in which many individuals generate and interact with complex flows. Motivated by animal groups on the move, here we explore how the locomotion of many bodies emerges from their flow-mediated interactions. Through experiments and simulations of arrays of flapping wings that propel within a collective wake, we discover distinct modes characterized by the group swimming speed and the spatial phase shift between trajectories of neighbouring wings. For identical flapping motions, slow and fast modes coexist and correspond to constructive and destructive wing�wake interactions. Simulations show that swimming in a group can enhance speed and save power, and we capture the key phenomena in a mathematical model based on memory or the storage and recollection of information in the flow field. These results also show that fluid dynamic interactions alone are sufficient to generate coherent collective locomotion, and thus might suggest new ways to characterize the role of flows in animal groups.

Multiscale polar theory of Microtubule and Motor-Protein Assemblies
by T. Gao, R. Blackwell, M. Glaser, M. Betterton, and M. Shelley
in Physical Review Letters 114, 048101 (2015)

Abstract: Microtubules and motor proteins are building blocks of self-organized subcellular biological structures such as the mitotic spindle and the centrosomal microtubule array. These same ingredients can form new �bioactive� liquid-crystalline fluids that are intrinsically out of equilibrium and which display complex flows and defect dynamics. It is not yet well understood how microscopic activity, which involves polarity dependent interactions between motor proteins and microtubules, yields such larger-scale dynamical structures. In our multiscale theory, Brownian dynamics simulations of polar microtubule ensembles driven by cross-linking motors allow us to study microscopic organization and stresses. Polarity sorting and crosslink relaxation emerge as two polar-specific sources of active destabilizing stress. On larger length scales, our continuum Doi-Onsager theory captures the hydrodynamic flows generated by polarity-dependent active stresses. The results connect local polar structure to flow structures and defect dynamics.

Transport and buckling dynamics of an elastic fiber in in a viscous cellular flow
By N. Quennouz, M. Shelley, O. du Roure, and A. Lindner
in Journal of Fluid Mechanics 769, 387-402 (2015).

Abstract: We study, using both experiment and theory, the coupling of transport and shape dynamics for elastomeric fibres moving through an inhomogeneous flow. The cellular flow, created electromagnetically in our experiment, comprises many identical cells of counter-rotating vortices, with a global flow geometry characterized by a backbone of stable and unstable manifolds connecting hyperbolic stagnation points. Our mathematical model is based upon slender-body theory for the Stokes equations, with the fibres modelled as inextensible elastica. Above a certain threshold of the control parameter, the elasto-viscous number, transport of fibres is mediated by their episodic buckling by compressive stagnation point flows, lending an effectively chaotic component to their dynamics. We use simulations of the model to construct phase diagrams of the fibre state (buckled or not) near stagnation points in terms of two variables that arise in characterizing the transport dynamics. We show that this reduced statistical description quantitatively captures our experimental observations. By carefully reproducing the experimental protocols and time scales of observation within our numerical simulations, we also quantitatively explain features of the measured buckling probability curve as a function of the effective flow forcing. Finally, we show within both experiment and simulation the existence of short and long time scales in the evolution of fibre conformation.


Theory of active suspensions
by D. Saintillan and M. Shelley
in Complex Fluids in Biological Systems, S. Spagnolie (ed.), Springer-Verlag (2015)

Abstract: Active suspensions, of which a bath of swimming microorganisms is a paradigmatic example, denote large collections of individual particles or macromolecules capable of converting fuel into mechanical work and microstructural stresses. Such systems, which have excited much research in the last decade, exhibit complex dynamical behaviors such as large-scale correlated motions and pattern formation due to hydrodynamic interactions. In this chapter, we summarize efforts to model these systems using particle simulations and continuum kinetic theories. After reviewing results from experiments and simulations, we present a general kinetic model for a suspension of self-propelled rod-like particles and discuss its stability and nonlinear dynamics. We then address extensions of this model that capture the effect of steric interactions in concentrated systems, the impact of confinement and interactions with boundaries, and the effect of the suspending medium rheology. Finally, we discuss new active systems such as those that involve the interactions of biopolymers with immersed motor proteins, and surface-bound suspensions of chemically-powered particles.