Surface tension poses especially severe difficulties in simulating the evolution of free-surface flows. The Laplace-Young (or Gibbs-Thompson) boundary condition introduces high-order, nonlinear and nonlocal terms, which give strong, time-dependent stability constraints on explicit time-stepping schemes.

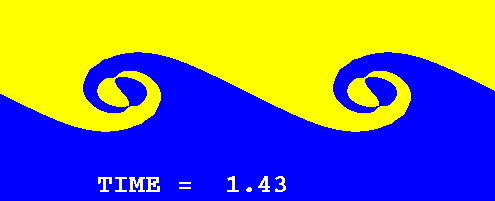
The figures below are from simulations of the effect of surface tension mixing of immiscible fluids by the classical Kelvin-Helmholtz instability. The numerical method is based upon a boundary integral formulation of the equations of motion and employs the Small-Scale Decomposition (SSD) to remove high-order time-stepping constraints. This has allowed the evolving flow to be computed with very high accuracy, and over much longer times than had been possible previously. Adaptive spatial grids methods have also been developed and employed within this methodology.

Click a box to see a short mpeg clip.

These figures show the results of numerical simulations of the mixing of two immiscible fluid through Kelvin-Helmholtz instability. Two horizontal spatial periods are shown. The top figure shows evolution from a single k=1 perturbation. The middle figure shows evolution from a perturbation primarily at k=3, with a slight subharmonic (k=1) contribution. The bottom figure shows evolution from initial data with randomly chosen amplitudes and phases.

The Weber number (We) measures the relative importance of inertial to surface tension forces; Here We=200, and there are many unstable scales along the dividing interface. Each simulation begins with a nearly flat interface, and typically ends with the disparate parts of the interface colliding, simultaneously forming corners. This is a "topological singularity", and implies the divergence of velocity gradients. This singularity is driven by the surface tension, and presumeably signals an incipient topological transition in the flow (a bubble forms).

These simulations were performed on a Cray C-90 and a dual processor Silicon Graphics Onyx RE2/R8000. Related simulations use the GMRES iterative method for rapid solution of associated integral equations (using SSD preconditioning), and the Fast Multipole Method (FMM) of Greengard & Rokhlin for rapid velocity evaluations. The animations were made on the SGI Onyx using OpenGL-based software. 

Accomplishments and Impact:

(i.) Surface tension is a central, but generally ill-understood, dynamical effect in multi-component fluid flows. It drives or mediates fluid mixing, bubble formation, determines length- and time-scales, etc. Numerical methods based upon the SSD allow the efficient and highly accurate solution of a nontrivial class of such free-surface problems, understanding the physics of which is important to the mission the Department of Energy. This new method is developed and implemented, for this and other problems, in

Removing the Stiffness from Interfacial Flows with Surface Tension
Journal of Computational Physics , Vol. 114, p. 312, 1994.
by T.Y. Hou (Caltech), J.S. Lowengrub (Minnesota), and M.J. Shelley
Download   gzipped postscript    PDF
 

The SSD has since been employed and extended by ourselves and other researchers to study many interfacial flow problems driven or mediated by a surface tension or energy. These include studies of the Kelvin-Helmholtz and Rayleigh-Taylor instabilities, water waves, and pattern formation and singularity development in Hele-Shaw flows. In the materials context, studies include pattern formation in directional solidification, the symmetric solidification model, Ostwald ripening with many inclusions (5000-10000), and the coarsening of elastic solids

(ii.) These simulations have revealed that a rich variety of behavior arises from a simple model of fluid mixing. For small Weber numbers, there are no unstable length-scales, and the flow is dispersively dominated. For intermediate Weber numbers, where there are only a few unstable length-scales, the interface forms elongating fingers of fluid that interpenetrate each fluid into the other. At larger Weber numbers, with many unstable scales, the interface rolls-up into a ``Kelvin-Helmholtz'' spiral, with its late evolution terminated by the collision of the interface with itself, forming at that instant bubbles of fluid at the core of the spiral. We study carefully this singular event (a ``topological'' or ``pinching'' singularity) using adaptive grid methods. The numerical techniques and simulational results are discussed in

The Long-Time Evolution of Vortex Sheets with Surface Tension
The Physics of Fluids , Vol. 9, p. 1933, 1997.
by T.Y. Hou (Caltech), J.S. Lowengrub (Minnesota), and M.J. Shelley
Download   gzipped postscript   PDF

This paper is cited in our being awarded the 1998 Frenkiel Award by the Division of Fluid Dynamics of the American Physical Society.

See also our review:  
Boundary Integral Methods for Multicomponent Materials and Multiphase Fluids,
(w. T. Hou and J. Lowengrub)
Journal of Computational Physics 169, p. 302 (2001).
Download PDF