Flexible/Moveable Bodies in Flowing Fluids
Modeling simple locomotors in Stokes flow
by Alex Kanevsky, Michael J. Shelley and Anna-Karin Tornberg
submitted (2008).
|
|
Abstract:
Motivated by the locomotion of flagellated micro-organisms and by
recent experiments of chemically driven nanomachines, we study the
dynamics of bodies of simple geometric shape that are propelled by
specified tangential surface stresses. We develop a mathematical
description of the body dynamics based on a mixed-type boundary
integral formulation. We also derive analytic axisymmetric solutions
for the case of a single locomoting sphere and ellipsoid based on
spherical and ellipsoidal harmonics, and compare our numerical results
to these. The hydrodynamic interactions between two spherical and
ellipsoidal swimmers in an infinite fluid are then simulated using
second-order
accurate spatial and temporal discretizations. We find that the
near-field interactions result in complex and interesting changes in
the locomotors’ orientations and trajectories. Stable as well as
unstable pairwise swimming motions are observed, similar to the recent
findings of Pooley
|
Peristaltic pumping and irreversibility of a Stokes viscoelastic fluid
by J. Teran, L. Fauci, and M. Shelley
submitted (2008).
|
|
Abstract:
Peristaltic pumping by wave-like contractions is a fundamental
biomechanical mechanism for fluid and material transport, and is used
in the esophagus, intestine, oviduct and ureter. While peristaltic
pumping of a Newtonian fluid is well understood, in many important
applications, as in the fluid dynamics of reproduction, the °uids
have non-Newtonian responses. Here, we present a numerical method for
simulating an Oldroyd-B fluid coupled to contractile, moving walls. A
MAC grid-based projection method is used for the fluid equations and an
immersed boundary method is used for coupling to a Lagrangian
representation of the deforming walls. We examine numerically the
peristaltic transport of a highly viscous Oldroyd-B fluid over a range
of Weissenberg numbers and peristalsis wave-lengths and amplitudes.
|
Instabilities and pattern formation in active particle suspensions:
Kinetic theory and continuum simulations
by D. Saintillan and M. Shelley, to appear in Physical Review Letters (2008).
|
|
Abstract:
We use
kinetic theory and non-linear continuum simulations to study the
collective dynamics in suspensions of self-propelled particles. The
stability of aligned suspensions is first analyzed, and we demonstrate
that such suspensions are always unstable to fluctuations, a result
that generalizes previous predictions by Simha and Ramaswamy (2002).
Isotropic suspensions are also considered, and it is shown that an
instability for the particle stress occurs in that case. Using
simulations, non-linear effects are investigated, and the long-time
behavior of the suspensions is observed to be characterized by the
formation of strong density fluctuations, resulting in efficient fluid
mixing.
|
The flapping states of a flag in an inviscid fluid: bistability and the
transition to chaos
by S. Alben and M. Shelley
Physical Review Letters 100, 074301
(2008).
|
|
Abstract:
We investigate the "flapping flag" instability through a model for an
inextensible flexible sheet in an inviscid 2D flow with a free vortex
sheet. We solve the fully-nonlinear dynamics numerically and find a
transition from a power spectrum dominated by discrete frequencies to
an apparently continuous spectrum of frequencies. We compute the linear
stability domain which agrees with previous approximate models in
scaling but differs by large multiplicative factors. We also find
hysteresis, in agreement with previous experiments.
|
Rotational dynamics of a superhelix towed in a Stokes fluid
by S. Jung, K. Mareck, L. Fauci, and M. Shelley
Physics of Fluids 19, 103105
(2007)
|
|
Abstract:
Motivated
by the intriguing motility of spirochetes of helically shaped bacteria
that screw through viscous fluids due to the action of internal
periplasmic flagella, we examine the fundamental fluid dynamics of
superhelices translating and rotating in a Stokes fluid. A superhelical
structure may be thought of as a helix whose axial centerline is not
straight, but also a helix. We examine the particular case in which
these two superimposed helices have different handedness, and employ a
combination of experimental, analytic, and computational methods to
determine the rotational velocity of superhelical bodies being towed
through a very viscous fluid. We find that the direction and rate of
the rotation of the body is a result of competition between the two
superimposed helices; for small axial helix amplitude, the body
dynamics is controlled by the short-pitched helix, while there is a
crossover at larger amplitude to control by the axial helix.We find far
better, and excellent, agreement of our experimental results with
numerical computations based upon the method of Regularized Stokeslets
than upon the predictions of classical resistive force theory.
|
Liquid crystal droplet production in a
microfluidic device
by B. Hamlington, B. Steinhaus, J. Feng, D. Link, A.-Q. Shen, and M. Shelley
in Liquid Chrystals 34, 861-870 (2007).
|
|
Abstract:
Liquid
crystal drops dispersed in a continuous phase of silicone oil are
generated with a narrow distribution in droplet size in microfluidic
devices both above and below the nematic-to-isotropic transition
temperature. Our experiments show that the surface properties of the
channels can be critical for droplet formation. We observe different
dynamics in liquid crystal droplet generation and coalescence, and
distinct droplet morphology on altering the microchannel surface
energy. This is explained by the thermodynamic description of the
wetting dynamics of the system. The effect of the nematic-to-isotropic
transition on the formation of liquid crystal droplets is also observed
and related to the capillary number. We also investigate how the
nematic droplet size varies with the flow rate ratio and compare this
behaviour with a Newtonian reference system. The effect of the defect
structures of the nematic liquid crystal can lead to distinctly
different scaling of droplet size in comparison with the Newtonian
system. When the nematic liquid crystal phase is stretched into a thin
filament before entering the orifice, different defect structures and
numbers of defect lines can introduce scatter in the drop size.
Capillary instabilities in thin nematic liquid crystal filament have an
additional contribution from anisotropic effects such as surface
gradients of bending stress, which can provide extra instability modes
compared with that of isotropic fluids.
|
Orientational
order and instabilities in suspensions of self-locomoting rods
by D. Saintillan and M. Shelley
in Physical Review Letters 99, 058102 (2007).
|
|
Abstract:
The orientational order and dynamics in suspensions of self-locomoting
slender rods are investigated numerically. In agreement with previous
theoretical predictions, nematic suspensions of swimming particles are
found to be unstable at long wavelengths as a result of hydrodynamic
fluctuations. Nevertheless, a local nematic ordering is shown to
persist over short length scales and to have a significant impact on
the mean swimming speed. Consequences of the large-scale orientational
disorder for particle dispersion are also discussed.
|
Emergence
of Singular Structures in Oldroyd-B Fluids
by Becca Thomases and M. Shelley
in Physics
of Fluids 19, 103103
(2007).
|
|
Abstract:
Numerical simulations reveal the formation of singular
structures in the polymer stress field of a viscoelastic
fluid modeled by the Oldroyd-B equations driven by
a simple body force. These singularities emerge exponentially in
time at hyperbolic stagnation points in the flow and their
algebraic structure depends critically on the Weissenberg number.
Beyond a first critical Weissenberg number the stress field approaches
a cusp singularity, and beyond a second critical Weissenberg number
the stress becomes unbounded exponentially in time. A local approximation
to the solution at the hyperbolic point is derived from
a simple ansatz, and there is excellent agreement between the
local solution and the simulations. Although the stress field becomes
unbounded for a sufficiently large Weissenberg number, the resultant forces
of stress grow subexponentially. Enforcing finite polymer chain lengths via
a FENE-P penalization appears to keep the stress bounded, but
a cusp singularity is still approached exponentially in time.
|
A
Stretch-Coil Transition and Transport of Fibers in Cellular Flows
by Y.-N. Young and M. Shelley
in Physical
Review Letters 99,
058303 (2007).
|
|
Abstract:
It is shown that a slender elastic fiber moving in a Stokesian fluid can
be susceptible to a buckling instability -- termed the "stretch-coil"
instability -- when moving in the neighborhood of a hyperbolic
stagnation point of the flow. When the stagnation point is
embedded in an extended cellular flow, it is found that immersed fibers
can move as random walkers across time-independent closed-streamline
flows. It is also found that the flow is segregated into
transport regions around hyperbolic stagnation points and their
manifolds, and closed entrapment regions around elliptic points.
|
Surface waves on a semitoroidal water ring
by Sungwhan Jung, Erica Kim, Michael Shelley and Jun Zhang
in Physics of Fluids 19, 058105 (2007).
|
|
Abstract:
We study the dynamics of surface waves on a semitoroidal ring of water that is excited by vertical
vibration. We create this specific fluid volume by patterning a glass plate with a hydrophobic
coating, which confines the fluid to a precise geometric region. To excite the system, the supporting
plate is vibrated up and down, thus accelerating and decelerating the fluid ring along its toroidal
axis. When the driving acceleration is sufficiently high, the surface develops a standing wave, and
at yet larger accelerations, a traveling wave emerges. We also explore frequency dependencies and
other geometric shapes of confinement.
|
Dynamics
of a Deformable Body in a Fast Flowing Soap Film
by S. Jung, K. Mareck, M. Shelley, and Jun Zhang
in Physical Review Letters 97, 134502 (2006).
|
Click on the image for a movie from the experiment |
Abstract:
We study the behavior of an elastic loop embedded in a flowing soap film. This
deformable loop is wetted into the film and is held fixed at a single
point against the oncoming flow. We interpret this system as a
two-dimensional flexible body interacting in a two-dimensional flow.
This coupled fluid-structure system shows bistability, with both
stationary and oscillatory states. In its stationary state, the loop
remains essentially motionless and its wake is a von
Ka┤rma┤n vortex
street. In its oscillatory state, the loop sheds two vortex dipoles, or
more complicated vortical structures, within each oscillation period.
We find that the oscillation frequency of the loop is linearly
proportional to the flow velocity, and that the measured Strouhal
numbers can be separated based on wake structure.
|
Periodic sedimentation in a Stokesian fluid
by S. Jung, S. E. Spagnolie, K. Parikh, M. Shelley, and A-K.
Tornberg
in Physical Review E 74, Rapid Communications, 035302
(2006)
|
Click on the image for a movie from the experiment |
Abstract:
We study the
sedimentation of two identical but nonspherical particles sedimenting
in a Stokesian fluid. Experiments and numerical simulations
reveal periodic orbits wherein
the bodies mutually induce an in-phase rotational motion accompanied by
periodic modulations of sedimentation
speed and separation distance. We term these “tumbling orbits” and
find that they appear over a broad range of body shapes.
|
Coherent Locomotion as an
Attracting State for a Free Flapping Body
by S. Alben and M. Shelley,
in Proceedings of the National
Academy of Sciences USA 102,
11163-11166 (2005).
|
Click on the image for movies referenced in this publication |
Abstract:
A common strategy for locomotion through a
fluid uses appendages, such as wings or fins, flapping perpendicularly
to the
direction of travel. This is in marked difference to strategies using
propellers
or screws, ciliary waves, or rowing with limbs or oars which explicitly
move fluid in the direction opposite to travel. Flapping locomotion is
also
never observed for microorganisms moving at low Reynolds number. To
understand
the nature of flapping locomotion we study numerically the dynamics of
a
simple body, flapped up and down within a viscous fluid and free to
move
horizontally. We show here that, at sufficiently large frequency
Reynolds
number, unidirectional locomotion emerges as an attracting state
for
an initially nonlocomoting body. Locomotion is generated in two stages:
first,
the fluid field loses symmetry by the classical von Karman instability;
and
second, precipitous interactions with vortical structures shed in
previous
flapping cycles push the body into locomotion. Body mass
and
slenderness play central and unexpected roles in each stage.
Conceptually,
this work demonstrates how locomotion can be transduced from the simple
oscillations of a body through an interaction with its fluid
environment.
|
Heavy flags undergo spontaneous
oscillations in flowing water
by M. Shelley, N. Vandenberghe, and J. Zhang,
in Physical Review Letters 94, 094302 (2005).
|
|
Abstract: By immersing a compliant yet self-supporting sheet
into flowing water, we study a heavy, stream-lined and elastic body
interacting with a fluid. We find that above a critical flow
velocity a sheet aligned with the flow begins to flap with a Strouhal
frequency consistent with animal locomotion. This transition is
subcritical. Our results agree qualitatively with a simple fluid
dynamical model that predicts linear instability at a critical flow
speed. Both experiment and theory emphasize the importance of
body inertia in overcoming the stabilizing effects of finite rigidity
and fluid drag.
|
Moore's Law and the Saffman-Taylor Instability
Petri Fast and Michael J. Shelley
Journal of Computational Physics 212, 1-5 (2005).
|
Click on the image for a movie from the experiment |
Abstract:
Ten years ago Hou, Lowengrub and Shelley published a state-of-the-art boundary
integral simulation of a classical viscous fingering problem,
the Saffman-Taylor instability. In terms of complexity and level of detail,
those computations are still among the most ramified and accurately computed
interfacial instability patterns that have appeared in the literature.
Since 1994, the computational power of a standard workstation has increased
a hundredfold as predicted by Moore's law.
The purpose of this Note is to consider Moore's law and its consequences
in computational science, and in particular,
its impact on studying the Saffman-Taylor instability.
We illustrate Moore's law and fast algorithms in action by presenting
the worlds largest viscous fingering simulation to date.
|
Stretch-flow of thin layers of
Newtonian liquids: Fingering patterns and lifting forces
by A. Lindner, D. Derks, and M. J. Shelley,
Physics of Fluids 17, 072107:1-13 (2005).
|
|
Abstract:
We study the stretch flow of a thin layer of Newtonian
liquid constrained between two circular plates. The evolution of the
interface of the originally circular bubble is studied when lifting one
of the plates at a constant velocity and the observed pattern is
related to the measured lifting force. By comparing experimental
results to numerical simulations using a Darcy's law model we can
account for the fully non-linear evolution of the observed fingering
pattern. One observes an initial destabilization of the interface by
growth of air fingers due to a Saffman Taylor like instability and then
a coarsening of the pattern towards a circular interface until
complete debonding of the two plates occurs. Numerical
simulations
reveal that when relating the observed patterns to the lifting force
not
only the number of fingers but also the amplitude of the fingering
growth
has to be taken into account. This is in agreement with the
experimental
observations.
|
Falling
Cards
by M. Jones and M. Shelley
Journal of Fluid Mechanics 540, 393-425 (2005).
|
|
Abstract:
In this study we consider the unsteady separated flow
of an inviscid fluid around a falling flat plate of small thickness and
high aspect ratio. The motion of the plate, which is initially released
from rest, is unknown in advance and is determined as part of the
solution. The flow solution is assumed two-dimensional and to consist
of a bound
vortex sheet coincident with the plate and two free vortex sheets that
emanate from each of the plate's two sharp edges. Throughout its
motion,
the plate continually sheds vorticity from each of its two sharp edges
and the unsteady Kutta condition, which states the fluid velocity must
be bounded everywhere, is applied at each edge. The coupled equations
of motion for the plate and its trailing vortex wake are derived and
are
shown to depend only on a modified Froude number.
|
A
Moving Overset Grid Method for Interface Dynamics applied to
Non-Newtonian Hele-Shaw Flow
by P. Fast and M. Shelley
Journal of Computational Physics 195, 117-142 (2004).
|
|
Abstract:
We present a novel moving overset
grid scheme for the accurate and efficient long-time simulation of an
air bubble displacing a non-Newtonian fluid in the prototypical thin
film device, the
Hele-Shaw cell. We use a two-dimensional generalization of Darcy's law
that
accounts for shear thinning of a non-Newtonian fluid. In the limit of
weak
shear thinning, the pressure is found from a ladder of two linear
elliptic boundary value problems, each to be solved in the whole fluid
domain. A
moving body fitted grid is used to resolve the flow near the interface,
while most of the fluid domain is covered with a fixed Cartesian grid.
Our use of body-conforming grids reduces grid anisotropy effects and
allows
the accurate modeling of boundary conditions.
|
Dynamics of Liquid Crystal Elastomers in Fluids:
A nonlocal model for liquid
crystal elastomers
by R. Ennis , L. Malacarne , P. Palffy-Muhoray and M.
Shelley
in Physical Review E 74, 061802 (2006).
|
|
Abstract:
We have developed a fully nonlocal model to describe the dynamic behavior of nematic liquid-crystal
elastomers. The free energy, incorporating both elastic and nematic contributions, is a function of the material
displacement vector and the orientational order parameter tensor. The free energy cost of spatial variations of
these order parameters is taken into account through nonlocal interactions rather than through the use of
gradient expansions. We also give an expression for the Rayleigh dissipation function. The equations of motion
for displacement and orientational order are obtained from the free energy and the dissipation function by the
use of a Lagrangian approach. We examine the free energy and the equations of motion in the limit of
long-wavelength and small-amplitude variations of the displacement and the orientational order parameter. We
compare our results with those in the literature. If the scalar order parameter is held fixed, we recover the usual
viscoelastic theory for nematic liquid crystals.
|
Fast Liquid-Crystal Elastomer
Swims into the Dark
by M. Comacho-Lopez, H. Finkelmann, P. Palffy-Muhoray, and M.
Shelley
In Nature Materials 3, 307-310 (2004).
|
|
Abstract: Liquid crystal elastomers (LCEs) are rubbers
whose constituent molecules are orientationally ordered. Their
salient feature is strong coupling between the orientational order and
mechanical strain. For example, stretching or otherwise deforming
an LCE sample changes the orientational order, which in turn changes
bulk properties such as birefringence and dielectric
susceptibility. Conversely, changing the orientational order
gives rise to internal stress, which
leads to strains that can change the shape of a sample. While
orientational order can be affected by changes in temperature and other
externally applied fields, light can also change the orientational
order via a number
of distinct processes. We demonstrate here that by dissolving azo
dyes into an LCE sample, its mechanical deformation in response to
visible
light becomes large and very fast. Light induced bending of more
than 60o has been observed on the timescale of tens of milliseconds;
this
is more than two orders of magnitude faster than previous
results.
Rapid light induced deformations allow LCE materials to interact with
their
environment in new and unexpected ways. We report here also the
astonishing observation that when light from above is shined on a
dye-doped
LCE sample floating on water, the LCE "swims" away from the light.
|
Computational Methods for Filamentary Objects in low Re
Flows:
|
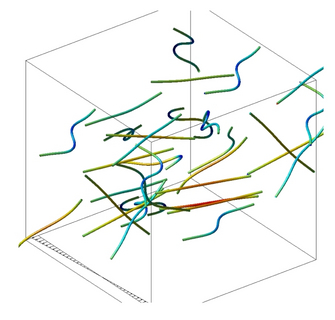
|
Flows in Nature and engineering often acquire their
interesting aspects by the presence in and interaction of the fluid
with immersed elastic objects. Fish, tree leaves, flagellae,
and rigid polymers all come to mind. A very important special
case is when the elastic bodies are microscopic and filamentary.
For example, flexible fibers make up the micro-structure of suspensions
that show strongly non-Newtonian bulk behavior, such as elasticity,
shear-thinning,
and normal stresses in shear flow. The figure to the right shows
a simulation of 25 flexible filaments undergoing interaction-induced
buckling
an an oscillatory shear show. These simulations are based on a
nonlocal
slender-body theory developed by Tornberg & Shelley.
Download Simulating
the dynamics and interactions of flexible fibers in Stokes flows, by
A.-K. Tornberg
and M.
Shelley, in J. of Computational Physics 196, 8-40
(2004).
See also
|
Flexible Bodies and Drag Reduction:
Experiment and theory from
the Courant Applied Mathematics
Lab:
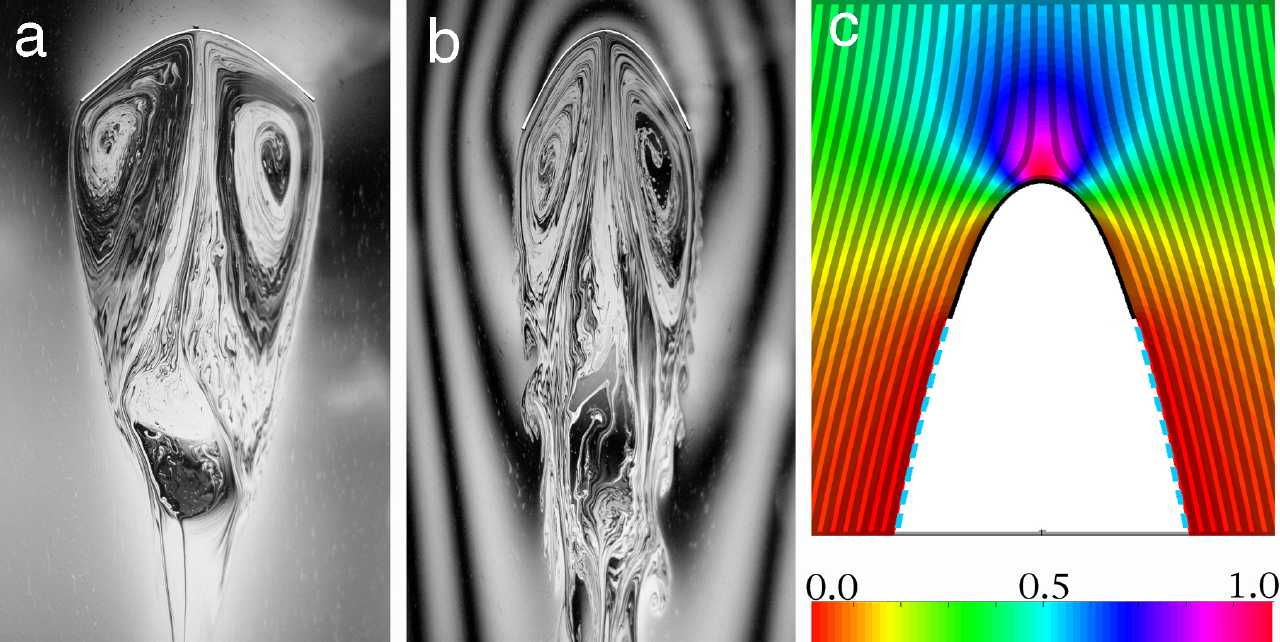 Nature abounds with organisms
utilizing body flexibility in order to survive in flowing fluids.
We study aspects of this using a length of fiber optic glass -- a
flexible body -- immersed in the the quasi- two-dimensional flow of a
running soap film. As the flow speed increases the shape of the
flexible body bends and becomes more and more streamlined -- the two
left panels -- and consequently the fluid drag on the body grows much
more slowly than if it were rigid. The rightmost figure shows the
numerical solution of a mathematical model of a flexible body deformed
by an surrounding flow and wake. This theory suggests an
emerging self-similarity in shape arising from a balance of fluid and
elastic forces at the tip. This self-similarity yields a new,
reduced drag law where drags grows as the 4/3's power, rather than the
square, of the flow velocity.
Nature abounds with organisms
utilizing body flexibility in order to survive in flowing fluids.
We study aspects of this using a length of fiber optic glass -- a
flexible body -- immersed in the the quasi- two-dimensional flow of a
running soap film. As the flow speed increases the shape of the
flexible body bends and becomes more and more streamlined -- the two
left panels -- and consequently the fluid drag on the body grows much
more slowly than if it were rigid. The rightmost figure shows the
numerical solution of a mathematical model of a flexible body deformed
by an surrounding flow and wake. This theory suggests an
emerging self-similarity in shape arising from a balance of fluid and
elastic forces at the tip. This self-similarity yields a new,
reduced drag law where drags grows as the 4/3's power, rather than the
square, of the flow velocity.
Download Drag
Reduction through Self-Similar Bending of a Flexible Body, by S. Alben, M. Shelley, and J. Zhang,
in Nature 420, 479-481 (2002).
Nature News and Views: Bend
and Survive by Victor Steinberg
Download How
Flexibility induces Streamlining in a Two-Dimensional Flow, by S. Alben, M. Shelley,
and J. Zhang, in Physics of Fluids 16,
1694-1713 (2004).
Flapping Flags:
An experiment from the Courant Institute Applied
Mathematics Lab:
 A flapping filament in a
quasi-two-dimensional soap film flow tunnel: The resulting motion is
similar to a flapping flag. We study the dynamics of a single
filament as well as multiple interacting filaments under the action
of an incoming laminar flow. Two stable states are observed in this
system. For more details click on the picture.
A flapping filament in a
quasi-two-dimensional soap film flow tunnel: The resulting motion is
similar to a flapping flag. We study the dynamics of a single
filament as well as multiple interacting filaments under the action
of an incoming laminar flow. Two stable states are observed in this
system. For more details click on the picture.
Download Flexible
filaments in a flowing soap film as a model for one-dimensional flags
in
a two-dimensional wind, by J. Zhang, S. Childress, A.
Libchaber and M.
Shelley, in Nature 408, 835-838 (2000).
Swinging Chains:

Time-exposure image of a swinging chain whose support is being
vertically oscillated (experiments performed at the Pritchard
Laboratory, Department of Mathematics, Penn State).
|

The numerical simulation of a swinging chain, using the
mathematical model of an inextensible many-linked chain.
|
Abstract: When shaken vertically, a hanging
chain displays a startling variety of distinct behaviors. We find
experimentally that a chain becomes unstable in tongue-like bands of
parameter space, either to swinging or rotating pendular states, or
to chaotic states. Mathematically, the chain dynamics are
described by a nonlinear wave equation. A linear stability
analysis predicts parametric instabilities within the well-known
resonance tongues; their boundaries agree very well with
experiment. Fully nonlinear
simulations of the 3D dynamics reproduce and elucidate many aspects
of the experimental observations. Experimentally, the chain is
also observed to tie knots in itself, drastically modifying its
dynamics.
Dynamic Patterns and Self-Knotting of a Driven
Hanging Chain, by Andrew
Belmonte, Shaden Eldakar, Michael Shelley,
and
Chris
Wiggins, in Physical Review Letters 87, 114301
(2001). Download
PDF
The pendular state:
Click here
to see an animation of the experiment
Click here
to see an animation of the simulation.
Some
entertaining publicity
Download our review of:
Boundary Integral Methods for Multicomponent Materials and Multiphase
Fluids,
by T. Hou, J. Lowengrub, and M. Shelley
in Journal of Computational Physics 169, 302-362 (2001).
(
|
|
Abstract:
We present a brief review of the application of boundary integral methods in two
dimensions to multicomponent fluid flows and multiphase problems in materials
science.We focus on the recent development and outcomes of methods which accurately
and efficiently include surface tension. In fluid flows, we examine the effects
of surface tension on the Kelvin┐Helmholtz and Rayleigh┐Taylor instabilities in inviscid
fluids, the generation of capillary waves on the free surface, and problems
in Hele-Shaw flows involving pattern formation through the Saffman┐Taylor instability,
pattern selection, and singularity formation. In materials science, we discuss
microstructure evolution in diffusional phase transformations, and the effects of the
competition between surface and elastic energies on microstructure morphology. A
common link between these different physical phenomena is the utility of an analysis
of the appropriate equations of motion at small spatial scales to develop accurate and
efficient time-stepping methods.
|
Other Research Descriptions and Downloads on:
 Nature abounds with organisms
utilizing body flexibility in order to survive in flowing fluids.
We study aspects of this using a length of fiber optic glass -- a
flexible body -- immersed in the the quasi- two-dimensional flow of a
running soap film. As the flow speed increases the shape of the
flexible body bends and becomes more and more streamlined -- the two
left panels -- and consequently the fluid drag on the body grows much
more slowly than if it were rigid. The rightmost figure shows the
numerical solution of a mathematical model of a flexible body deformed
by an surrounding flow and wake. This theory suggests an
emerging self-similarity in shape arising from a balance of fluid and
elastic forces at the tip. This self-similarity yields a new,
reduced drag law where drags grows as the 4/3's power, rather than the
square, of the flow velocity.
Nature abounds with organisms
utilizing body flexibility in order to survive in flowing fluids.
We study aspects of this using a length of fiber optic glass -- a
flexible body -- immersed in the the quasi- two-dimensional flow of a
running soap film. As the flow speed increases the shape of the
flexible body bends and becomes more and more streamlined -- the two
left panels -- and consequently the fluid drag on the body grows much
more slowly than if it were rigid. The rightmost figure shows the
numerical solution of a mathematical model of a flexible body deformed
by an surrounding flow and wake. This theory suggests an
emerging self-similarity in shape arising from a balance of fluid and
elastic forces at the tip. This self-similarity yields a new,
reduced drag law where drags grows as the 4/3's power, rather than the
square, of the flow velocity. A flapping filament in a
quasi-two-dimensional soap film flow tunnel: The resulting motion is
similar to a flapping flag. We study the dynamics of a single
filament as well as multiple interacting filaments under the action
of an incoming laminar flow. Two stable states are observed in this
system. For more details click on the picture.
A flapping filament in a
quasi-two-dimensional soap film flow tunnel: The resulting motion is
similar to a flapping flag. We study the dynamics of a single
filament as well as multiple interacting filaments under the action
of an incoming laminar flow. Two stable states are observed in this
system. For more details click on the picture.

